Beer’s Law, Part III: Demystifying the Absorptivity

In my previous two columns I introduced you to Beer’s Law. In the first column (1), we examined the Beer’s Law equation and how it relates light absorbance to concentration, and how to use Beer’s Law to determine cannabinoid and terpene amounts in cannabis samples. In the second Beer’s Law column (2), I introduced the idea of totally inelastic collisions between photons and molecules and used that picture to derive Beer’s Law mathematically. We then discussed the limitations of Beer’s Law. Here in part III, we will finally come clean about what the absorptivity truly is, and how it impacts how we can use Beer’s Law.

Review

Beer’s Law

Beer’s Law allows spectroscopy to be quantitative because it relates the amount of light absorbed by a sample to the concentration of chemical species in that sample (1,2). Beer’s Law has the following form:

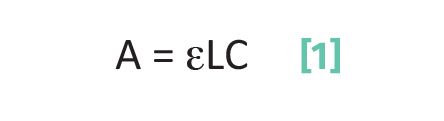
where A is the absorbance; ε is the absorptivity; L is the pathlength; and C is the concentration.

By rearranging Beer’s Law, we can see how to solve for the concentration of an analyte in an unknown sample as such:

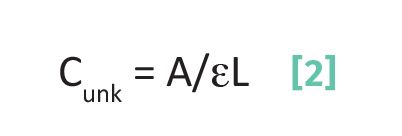
where C_unk is the concentration of analyte in an unknown sample.

Thus, measuring a concentration in a sample using Beer’s Law is a matter of measuring the absorbance of the sample, and knowing the product of the absorptivity and pathlength, εL. This product is obtained via the calibration process. Beer’s Law has the same form as the equation for a straight line as such (3):

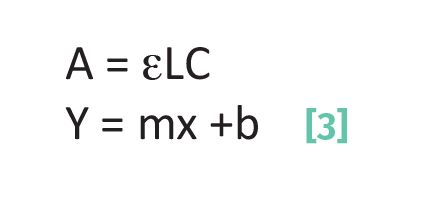
where Y is the y-axis data value; m is the slope of a line; x is the x-axis data value; and b is the y-intercept.

To obtain a Beer’s Law calibration, the absorbances of standard samples containing known amounts of the analyte are measured. Then, the measured absorbances for the standards are plotted on the y-axis, and the known analyte concentrations on the x-axis. If the sample system being analyzed obeys Beer’s Law, this plot will be a straight line whose slope is εL, the quantity we need to use along with Equation 2 to determine concentrations of analyte in unknown samples.

The Absorptivity

Recall (1,2) that the absorptivity is the proportionality constant between absorbance and concentration. It is a fundamental physical constant for a pure molecule at a given wavenumber of absorbance, temperature, and pressure. For example, the absorptivity of pure liquid water at 1 atmosphere of pressure, 25 °C, and at 1630 cm^-1 is a fundamental physical constant of the water molecule. The absorptivity changes from wavenumber to wavenumber for a given molecule. Thus, the absorptivity of water at 1630 cm^-1 is different than at 1000 cm^-1. Different molecules typically have different absorptivities at the same wavenumber. For example, the absorptivity of water and acetone at 1630 cm-1 are different. Lastly, in general, different molecules have different absorptivities at different wavenumbers. Thus, the absorptivity of water at 1630 cm^-1 is different than that of acetone at 1715 cm^-1.

Beer’s Law Units

Since the absorbance is a unitless quantity, when the units of the absorptivity, pathlength, and concentration are multiplied together they must cancel out. How does this happen? The units of concentration are in moles/volume. Remember that volume has units of width x height x length, which is length cubed, or L³. Which means the units of concentration are moles/L³. The units of the pathlength are simply length or L. When we multiply the units out in Beer’s Law the results are seen in Equation 4.

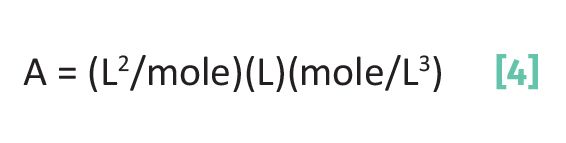
For the absorbance to be a unitless quantity the absorptivity must have units of L²/mole. These units may seem physically counterintuitive. Length² is an area, and a mole measures number of molecules. How can a collection of molecules have an area? As promised previously (1,2), all will now be revealed about the mysterious absorptivity.

Absorptivity: The Rest of the Story

Recall from the last column (2) that the absorbance process is the result of what are called totally inelastic collisions between photons and molecules. In a totally inelastic collision, all the energy in the photon is deposited into the molecule, and the photon ceases to exist. The resultant decrease in light intensity as measured by a spectrometer gives rise to an absorbance peak in the sample’s spectrum. When we derived Beer’s Law (2) we calculated the total number of collisions as being

where I is the light intensity in slab dL; C is the concentration; dL is an infinitesimally thin slab of sample and where I represents the number of photons in dL, and C represents the number of molecules in dL.

Now, only a fraction of collisions will be of the totally inelastic variety. As I said previously (2), the absorptivity represents the fraction of all collisions that are totally inelastic.

To summarize this is what we know about the absorptivity:

1. It is the proportionality constant between absorbance and concentration.
2. It is a fundamental physical constant of a pure molecule at a given wavenumber, temperature,
   and pressure.
3. It has units of length²/mole.
4. It represents the fraction of photon molecule collisions that are totally inelastic.

I could have added a fifth thing to this list, which would be the quantum mechanical definition of the absorptivity derived from things such as energy levels, wavefunctions, and wavefunction overlap (4). However, I am feeling merciful and will spare you the math behind that. How on earth can one simple physical constant have so many aspects to it? That’s what we are here to talk about.

Throwing Baseballs at Barns

How can something with units of length2/mole represent a fraction of totally inelastic collisions? As it turns out, characterizing collisions between microscopic particles is something physicists have been studying for a long time. For example, if you have heard about atom smashers and particle accelerators, this is what I am talking about (5). As it turns out, photon-molecule collisions are simply a subset of the types of collisions that physicists already understand.

One thing particle physicists are interested in is the probability that two particles will collide when they encounter each other. A unit that physicists use to describe the probability of two particles colliding is called the barn, which is defined as 1x10^-24 cm² (6). What on earth does this unit mean? Did you ever hear a baseball pitcher described as “not being able to hit the broad side of a barn?” Well, that is where the unit is derived from. I can relate to not being able to hit the broad side of a barn since my statistics from my Little League career as a starting pitcher are 1 1/3 innings pitched with 1 strikeout, 2 walks, 3 hits, and 4 earned runs. Needless to say, I was transferred back to right field after that performance.

At any rate, Figure 1 shows me trying to hit the broad side of a barn with a baseball.

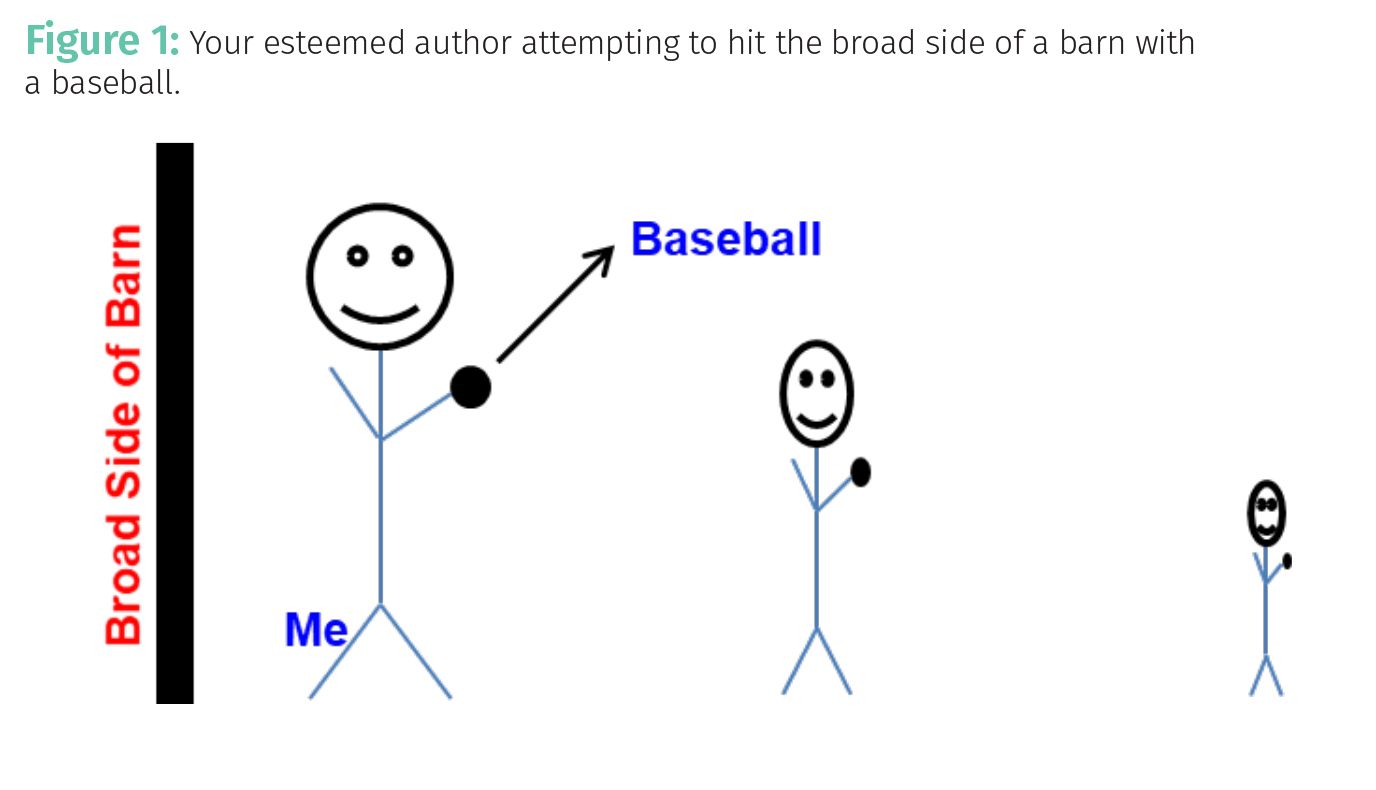
Note that when I stand close to the barn, as seen to the left in the figure, the barn appears large in area to me, and the probability of my hitting it is large. If I step back several paces, the side of the barn appears smaller to me than before and the probability of hitting it goes down. If I step back a long distance, as seen to the right in the figure, the area of the barn appears quite small to me, and my probability of hitting it is very low. Now, the units of area are length². So, the probability of my hitting the barn is determined by the apparent area of the barn from where I am standing. Thus, this is how probability has units of area.

Now, remember from above that the units of the absorptivity are length²/mole, or in other words probability/mole. Now, not to belabor the point, but a mole contains Avogadro’s number, or 6.02x10²³,molecules (Avogadro constant [7]), or 6.02x10²³ molecules/mole. If we divide the absorptivity in units of length2/mole by Avogadro’s number in units of molecules/mole, we obtain a quantity that is in units length²/molecule or probability/molecule. We obtain a quantity I will call the molecular absorptivity.

What the molecular absorptivity measures is the apparent area of a molecule from the point of view of a photon. If the molecule looks large to the photon, the probability of a totally inelastic collision will be high, and the absorptivity will be large. On the other hand, if the molecule looks small to the photon, the probability of a totally inelastic collision and hence the absorptivity will be small.

We stated above that the absorptivity describes the fraction of all collisions that are totally inelastic. How is this a probability? Let’s assume the fraction of totally inelastic collisions is 1/5 or 20%. This means that 20% of all collisions, or 1 in 5, are totally inelastic. This also means the odds of a collision occurring are 1 in 5, and that, folks, is a probability. So, our idea of the absorptivity describing the fraction of totally inelastic collisions is consistent with the units of the absorptivity being a probability.

Variables that Effect the Absorptivity

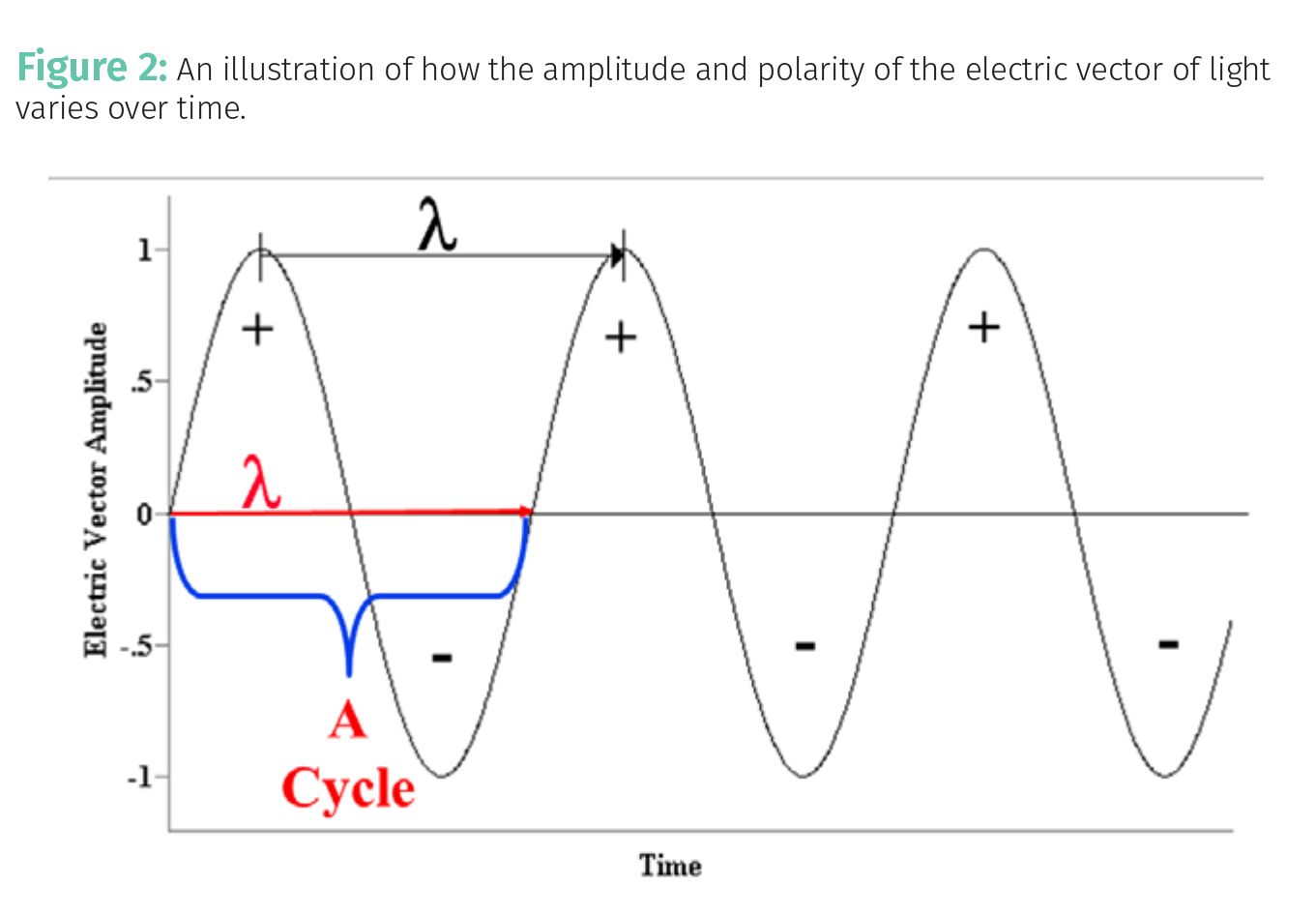
If the absorptivity describes how big a molecule looks from the point of view of a photon, what things will affect this quantity? Remember that light contains an electric vector (2) whose polarity varies over time from positive to negative to positive as illustrated in Figure 2.

The electronic structure of a molecule is simply a description of where the electrons and nuclei are located most of the time. The electronic structure of carbon dioxide is seen in Figure 3.

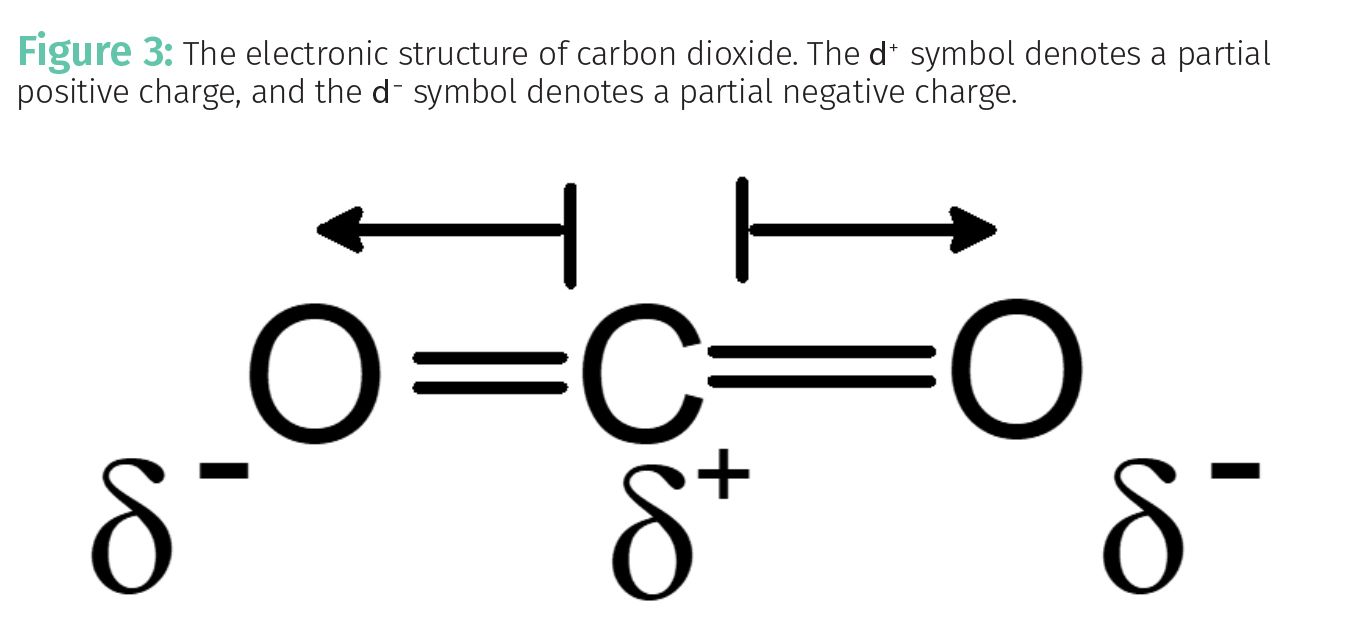
Because of the electronegativity differences between oxygen and carbon, the electrons in the carbon-oxygen bonds spend most of their time near the oxygen. This results in a partial positive charge, d⁺, forming on the carbon atom, and a partial negative charge, d⁻, forming on the oxygen atoms.

To tie this all together, it is the interaction between the electric vector and the electronic structure of the molecule that causes absorbance to take place. If the interaction is strong the molecule will appear “large” to the photon, the probability of a totally inelastic collision will be large, and hence the absorptivity will be large. Alternatively, if the interaction between the electric vector and the electronic structure of the molecule is weak, the molecule will appear “small” to the photon and hence the probability of a totally inelastic collision will be small as will be the absorptivity.

What variables then affect a molecule’s electronic structure and hence its absorptivity? One thing is intermolecular interactions; the strength of the interactions between a molecule and its neighboring molecules. An example of intermolecular interactions, the hydrogen bonding between water molecules, is seen in Figure 4.

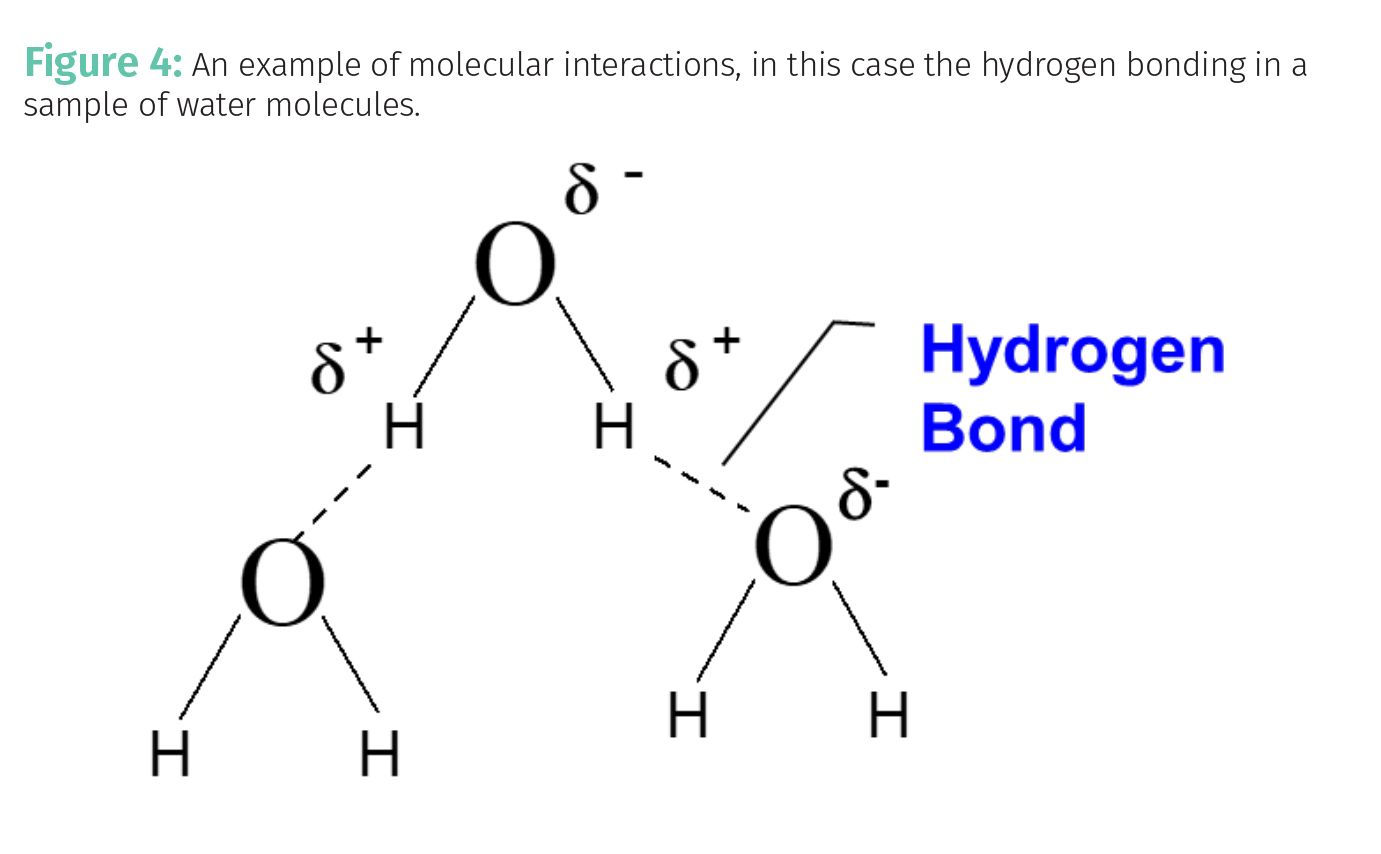
In Figure 4 the electronegativity difference between oxygen and hydrogen means the O-H bonds are polar and contain partial positive and negative charges. The positive hydrogen on one water molecule will coordinate with the negative oxygen on a neighboring molecule forming a strong intermolecular interaction called a hydrogen bond.

What variables then affect intermolecular interactions and hence the absorptivity?

1. Pressure: Imagine a sample of water vapor. At low pressure the water molecules are relatively far apart, and the intermolecular interactions will be weak. At higher pressure the molecules are forced closer together, the intermolecular interactions are stronger, and hence the absorptivity will change.
2. Temperature: Again consider a sample of water vapor. Temperature is a measure of the average kinetic energy of a collection of molecules (3). When molecules collide, their electronic structures are scrambled by the impact. At low temperature the water molecules will be moving slowly, and their collisions will be few and weak. At higher temperatures the collisions will be many and strong, which is why temperature affects the absorptivity.
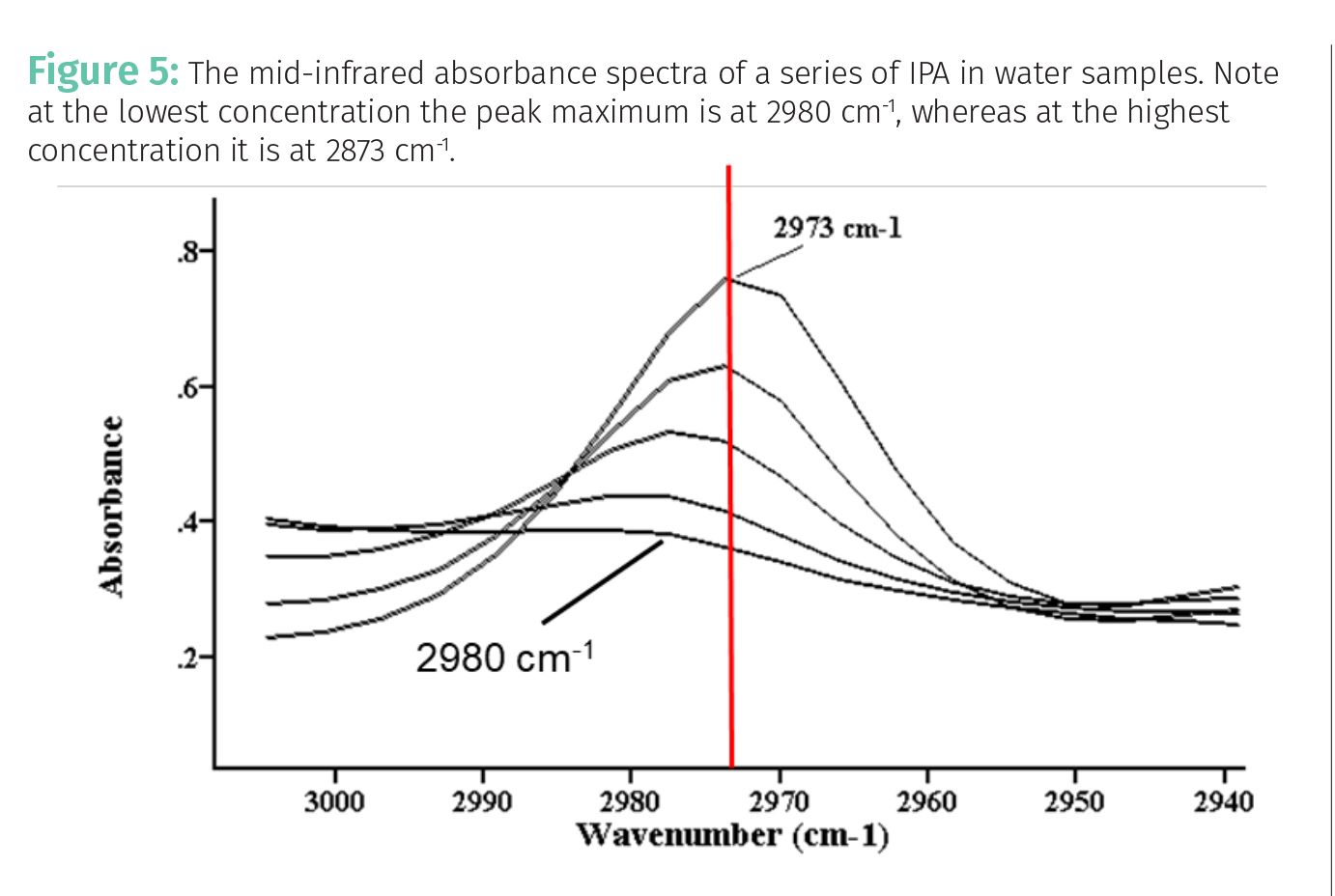
3. Concentration: Imagine a solution of isopropyl (rubbing) alcohol (IPA) in water. In pure IPA the nature of the intermolecular interactions will be different than in say, a solution of 10% IPA in water. This is illustrated in Figure 5, which shows the mid-infrared absorbance spectra of a series of five IPA in water samples, with the IPA concentration varying from 10% to 70%. These same spectra were seen in a previous column when we talked about calibration with Beer’s Law (1). Note that at the lowest concentration, where the peak is smallest, the peak top is at 2980 cm^-1. However, at the highest concentration, where the peak is largest, the peak top is at 2973 cm-1. This change in the position of the peak top is due to the absorptivity of IPA changing with concentration. We have seen previously that this data yielded an excellent calibration (1). Thus, if the change in absorptivity with concentration is small, Beer’s Law can still work.
4. Composition: Imagine a solution of 50% IPA in water, and a 50% solution of IPA in vinegar, which contains acetic acid. As you may know, pH is a measure of acidity and basicity, where 7 is neutral and lower numbers mean more acidic (8). The pH of the IPA/water solution is going be right around neutral or 7. The pH of the IPA/vinegar solution will be acidic and about 3. In both these cases, the concentration of IPA is the same, but the chemical composition of these two samples is different. The intermolecular interactions between IPA and the water molecules will be different than between IPA and the acetic acid molecules. This means the absorptivity of IPA in these two different solutions will be different, and its spectra will be different. This means composition affects the absorptivity, and since pH determines molecular composition, it too affects the absorptivity.

To summarize then, the variables of pressure, temperature, concentration, composition, and pH all affect intermolecular interactions, and hence electronic structure. This in turn will affect how light interacts with a molecule, hence the absorptivity. The dependence of the absorptivity on these variables is why when we say that the absorptivity is a constant for a pure substance, temperature, pressure, and other conditions must be specified.

Analytical chemists sometimes define a sample’s matrix as its physical and chemical condition, including things like temperature, pressure, concentration, composition, and pH. Another way of summarizing the bullet points above is that the absorptivity is matrix sensitive, which means Beer’s Law calibrations are matrix sensitive. Therefore, spectrometers can only be used to analyze things for which they have been calibrated (which is true of all analytical instruments). In my career with Big Sur Scientific, where we sell mid-infrared-based cannabis analyzers (9) I have had people complain about their analyzer because they used it to analyze pizza and yet it gave a total tetrahydrocannabinol (THC) reading. This is because the instrument can only accurately analyze sample types for which it has been calibrated, and if you use it to analyze samples for which it is not calibrated you will get nonsensical results. The matrix sensitivity of Beer’s Law is also why when you use a spectroscopy-based cannabis analyzer there are specific sample type choices such as cannabis, hemp, medium-chain triglyceride (MCT) oil tinctures, and so forth. It is up to the user to make sure the sample type chosen in the software matches the sample type actually being analyzed so that the correct calibrations are applied, and accurate results are obtained. Again, if the sample type chosen in the software does not match the sample type analyzed, inaccurate results will be obtained.

So, there you are, the rest of the story on the absorptivity. I bet you never guessed so there was so much science behind such a simple proportionality constant.

Conclusions

We reviewed Beer’s Law and how it is used to determine concentrations of molecules in unknown samples. We then reviewed what we knew about the absorptivity, including that it has units of length²/area. Using the example of me trying to throw a baseball at a barn, we showed that the idea of the absorptivity describing the probability of totally inelastic photon-molecule collisions makes sense. Then, using the concepts of the electric vector of light, molecular electronic structure, and intermolecular interactions we found that the absorptivity is affected by the variables of pressure, temperature, concentration, composition, and pH. This means that the absorptivity is matrix sensitive, which means we can only use spectroscopy to quantitate sample types for which we have a calibration.

References

1. B. Smith, Cannabis Science and Technology 5(2), 10-13 (2022).
2. B. Smith, Cannabis Science and Technology 5(3), 10-14 (2022).
3. Brian C. Smith, Quantitative Spectroscopy: Theory and Practice (Elsevier, Boston, Massachusetts, 2002).
4. J. Michael Hollas, Modern Spectroscopy 4th Edition (Wiley, New York, New York, 2013).
5. https://en.wikipedia.org/wiki/Particle_accelerator.
6. https://en.wikipedia.org/wiki/Barn_(unit).
7. https://en.wikipedia.org/wiki/Avogadro_constant
8. https://en.wikipedia.org/wiki/PH
9. http://www.bigsurscientific.com

About The Columnist

Brian C. Smith, PhD, is Founder, CEO, and Chief Technical Officer of Big Sur Scientific.

He is the inventor of the BSS series of patented mid-infrared based cannabis analyzers.

Dr. Smith has done pioneering research and published numerous peer-reviewed papers on the application of mid-infrared spectroscopy to cannabis analysis, and sits on the editorial board of Cannabis Science and Technology®. He has worked as a laboratory director for a cannabis extractor, as an analytical chemist for Waters Associates and PerkinElmer, and as an analytical instrument salesperson. He has more than 30 years of experience in chemical analysis and has written three books on the subject. Dr. Smith earned his PhD on physical chemistry from Dartmouth College. Direct correspondence to: brian@bigsurscientific.com

About The Columnist

B. Smith, Cannabis Science and Technology® Vol. 5(4), 8-15 (2022).