Chromatographic Theory, Part VI: Peak Widths, Band Spreading, and Plate Number

The last column dealt with measuring peak widths and using them in separation quality calculations, but what causes chromatographic peaks to have different widths? One of the culprits is band spreading, which is caused by diffusion. In this column, we discuss diffusion and see how a quantity called plate number is used to measure the amount of band spreading.

Peak Widths and Chromatographic Resolution: A Review

In my last column (1) we discovered that in addition to peak positions chromatographic features have peak widths. The technique we chose to measure peak widths was the triangulation method (1) seen in Figure 1.

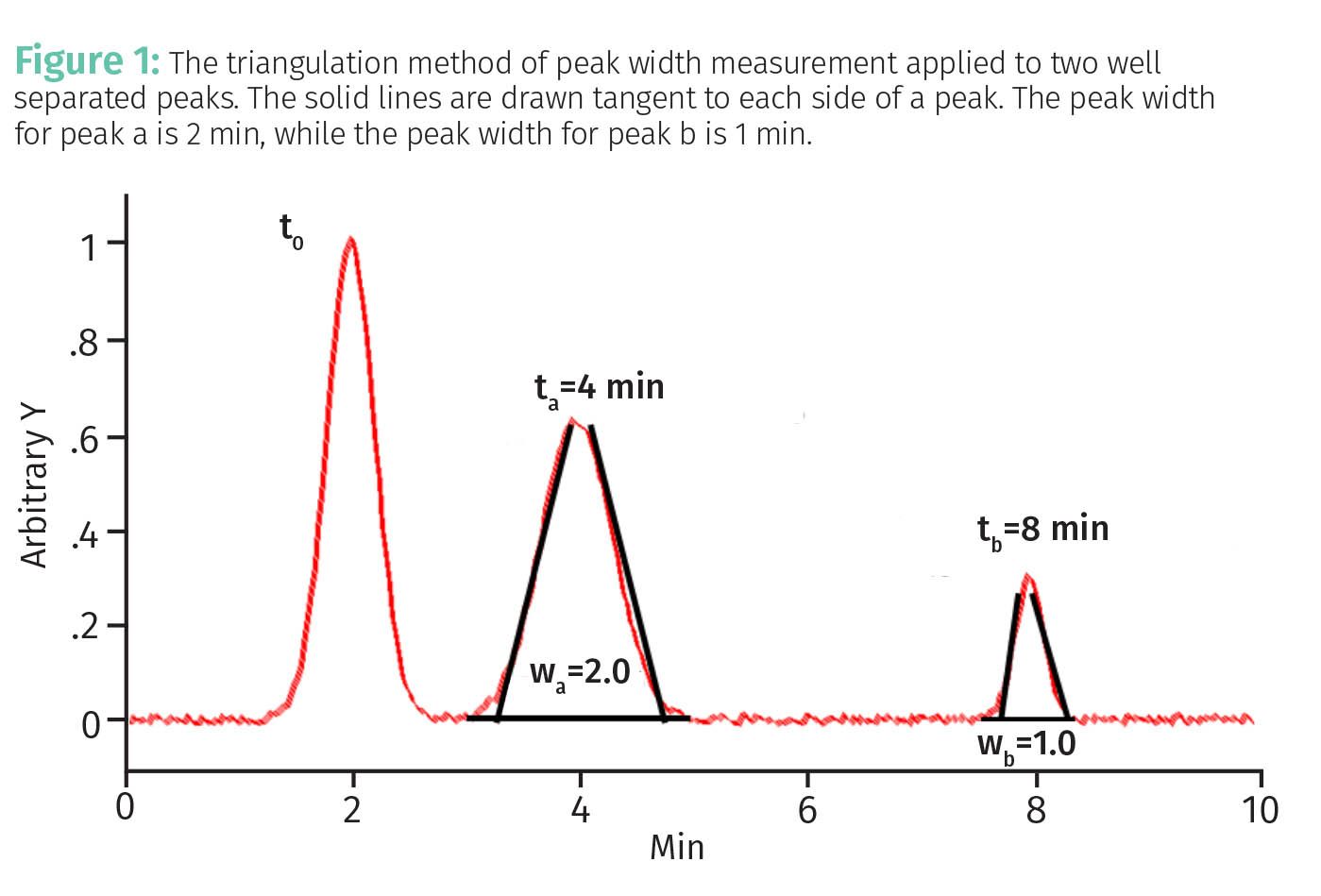
In this method a baseline is drawn under a peak, and then lines are drawn along the sides of the peak that intersect with the baseline to form two baseline endpoints. The width of the peak is measured between the two baseline endpoints to give the triangulated peak width. Using this method in Figure 1 the peak width w_a for peak a is 2 min, whereas the peak width w_b for peak b is 1 min.

For the chromatogram seen in Figure 1, the peak widths do not pose a problem because the peaks are baseline separated, that is, there is flat baseline between them. However, as we saw in the last installment of this series (1), excessively wide peaks affect separation quality as seen in Figure 2.

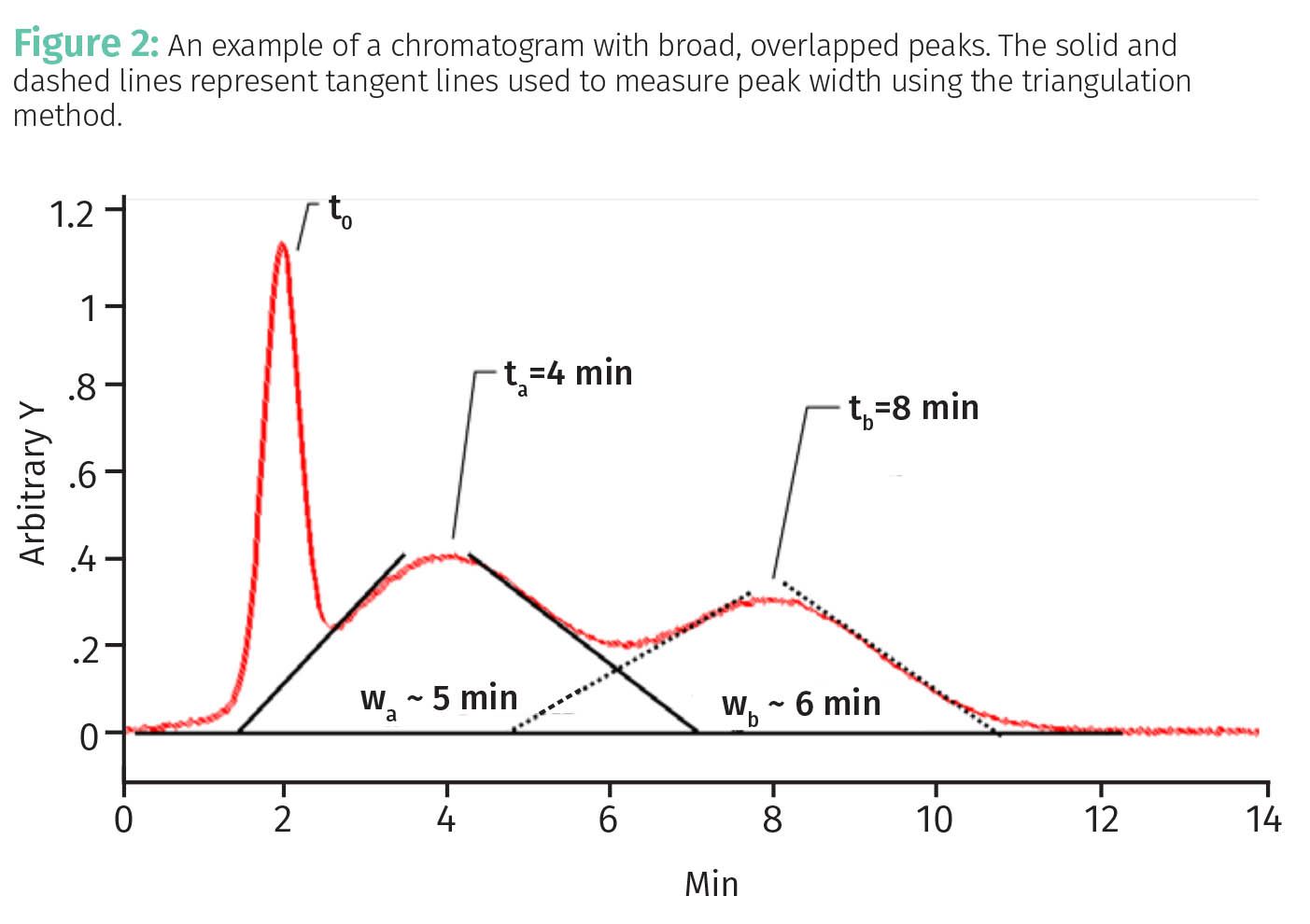
The retention times for peaks a and b in Figures 1 and 2 are the same, namely 4 min and 8 min, respectively, but in Figure 2 the separation is of poor quality because the peaks are overlapped due to excessive peak width. We can see this by comparing the widths of these peaks in the two figures. Peak a is 2 min wide in Figure 1 and 5 min wide in Figure 2, while peak b is 1 min wide in Figure 1 and 6 min wide in Figure 2.

The challenge we faced in the last column (1) was deriving a measure of chromatographic separation quality that took peak width into account. We found that this quantity is called the chromatographic resolution and is defined in Equation 1.

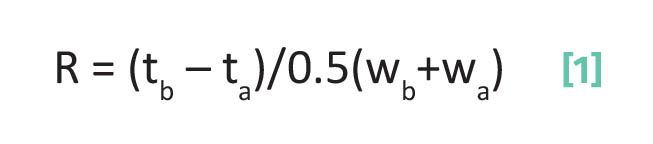
where R is the resolution, t_b is the elution time for peak b, t_a is the elution time for peak a, w_a is the width of peak a, w_b is the width of peak b, and with t_b > t_a.

The units in the numerator and denominator are both time, which means R is unitless. Note in the numerator of Equation 1 that the elution times for two chromatographic peaks are subtracted from each other. The stipulation that t_b be greater than t_a exists so that the numerator, and hence R, have a positive value. The denominator in Equation 5 is the average of the two peak widths. Because of this, as average peak width goes up R goes down. That is, the broader the chromatographic peaks the worse the quality of a separation and the lower the R value. Using Equation 1, the separation in Figure 1 has a resolution of 2.67, whereas the separation in Figure 2 has a resolution of 0.73. Baseline separated peaks have an R value of 1.5 or greater, which is always our goal when developing separation methods.

What Causes Broadened Peaks?

I refer you to the literature (2-4) for more details and background on the following discussion.

Figure 3 illustrates in red a slug or band of analyte after it has been injected at the head of a chromatographic column. The analyte band traverses the column from left to right. Note that upon injection at time T = 0 the analyte has adsorbed and the band has a width w_a. You can think of the red bars in Figure 3 as locations where the analyte is adsorbed on the stationary phase, and the white spaces as locations where the analyte is in the mobile phase.

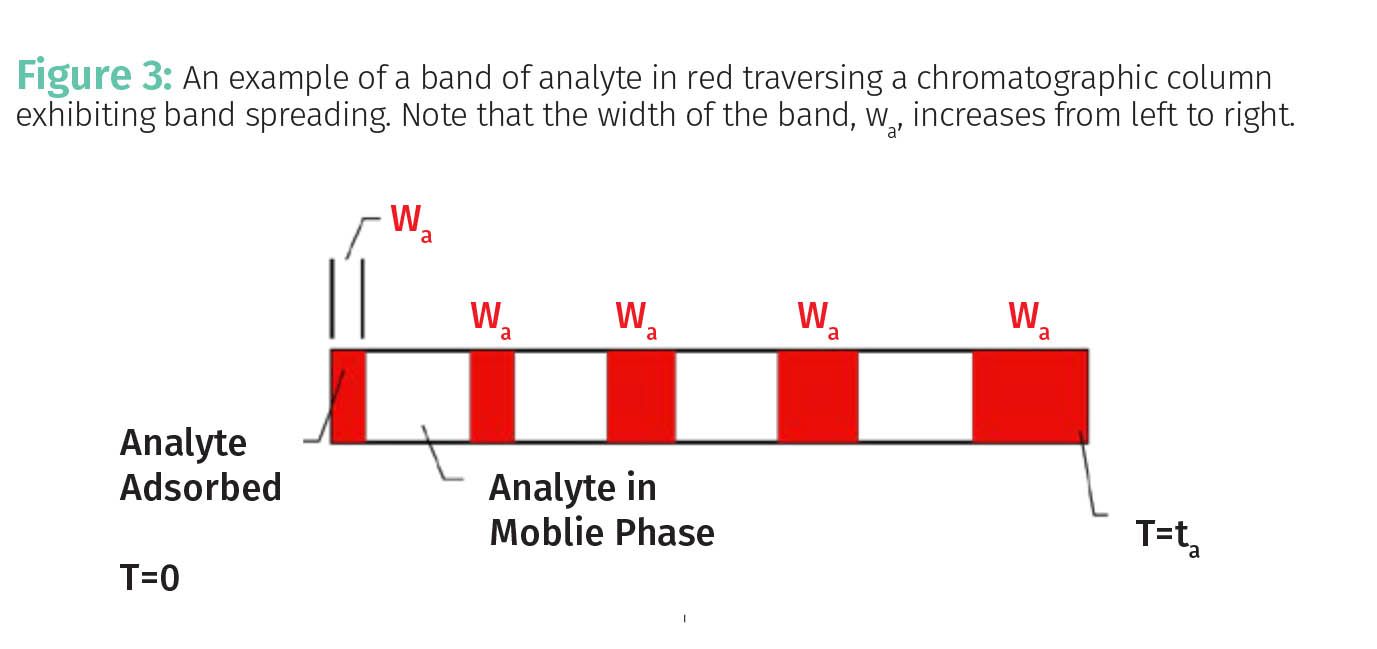
Note that there are five spots along the column where the analyte adsorbs, and as the analyte band traverses the column it gets wider and wider. What’s going on here? A phenomenon called band spreading caused by the process of diffusion (5). Diffusion occurs when chemical species spontaneously move from areas of higher concentration to areas of lower concentration. You can observe diffusion when you put cream in your morning coffee. The slug of cream doesn’t just sit there, you can see it move throughout your coffee cup. Note that the longer you watch the cream the more diffusion takes place. Stirring the coffee and cream mixture with your spoon speeds the process of diffusion, but left to its own devices for long enough the cream will infiltrate all parts of your coffee cup until the concentration of cream is the same everywhere.

Diffusion happens to analytes in chromatographic columns. When an analyte is adsorbed on the stationary phase it does not diffuse because it is held fast. However, when the analyte is in the mobile phase, such as in the white areas in Figure 3, the analyte molecules will diffuse along the longitudinal axis of the column broadening out the band and giving it increased peak width. That is why each red band in Figure 3 is wider and wider as it moves from left to right down the column until it elutes at retention time ta. Like the cream in your coffee, the more time your analyte spends in solution, the more it will diffuse and the more widespread the distribution of the analyte. The upshot here is that the more time your analyte spends in the mobile phase, the more diffusion will take place and the broader your peak will become.

To get the narrowest peaks then and to optimize separations you want to choose a method that minimizes the time your analyte spends in the mobile phase. Recall (6) that the elution time of an analyte depends upon the fraction of time an analyte spends adsorbed on the stationary phase as given by Equation 2:

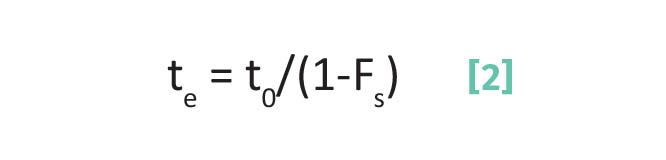
where t_e is the elution time, t₀ is the void time in minutes, and F_s is the fraction of time an analyte spends adsorbed on the stationary phase.

Also recall (7) that the total time a molecule spends in a column is simply the sum of the time it is adsorbed on the stationary phase and the time it is in the mobile phase as given by Equation 3:

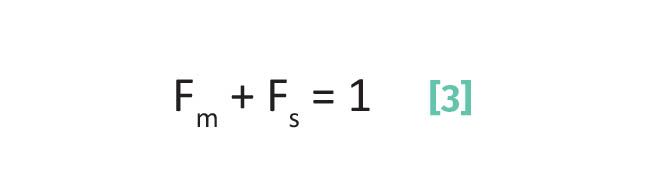
where F_m is the fraction of time an analyte spends in mobile phase and F_s is the fraction of time an analyte spends in the stationary phase.

While the analyte is in the column it is either in the mobile or stationary phases, so the fractions in Equation 3 add up to 1.

We said above that the more time the analyte spends in the mobile phase the more diffusion can take place and the broader our peak is when it elutes from the chromatographic column. In other words, the larger F_m is the broader our band will be.

Measuring Band Spreading: The Plate Number

Given the above then our measure of band spreading must be related to F_m somehow. To figure this out consider Figure 4.

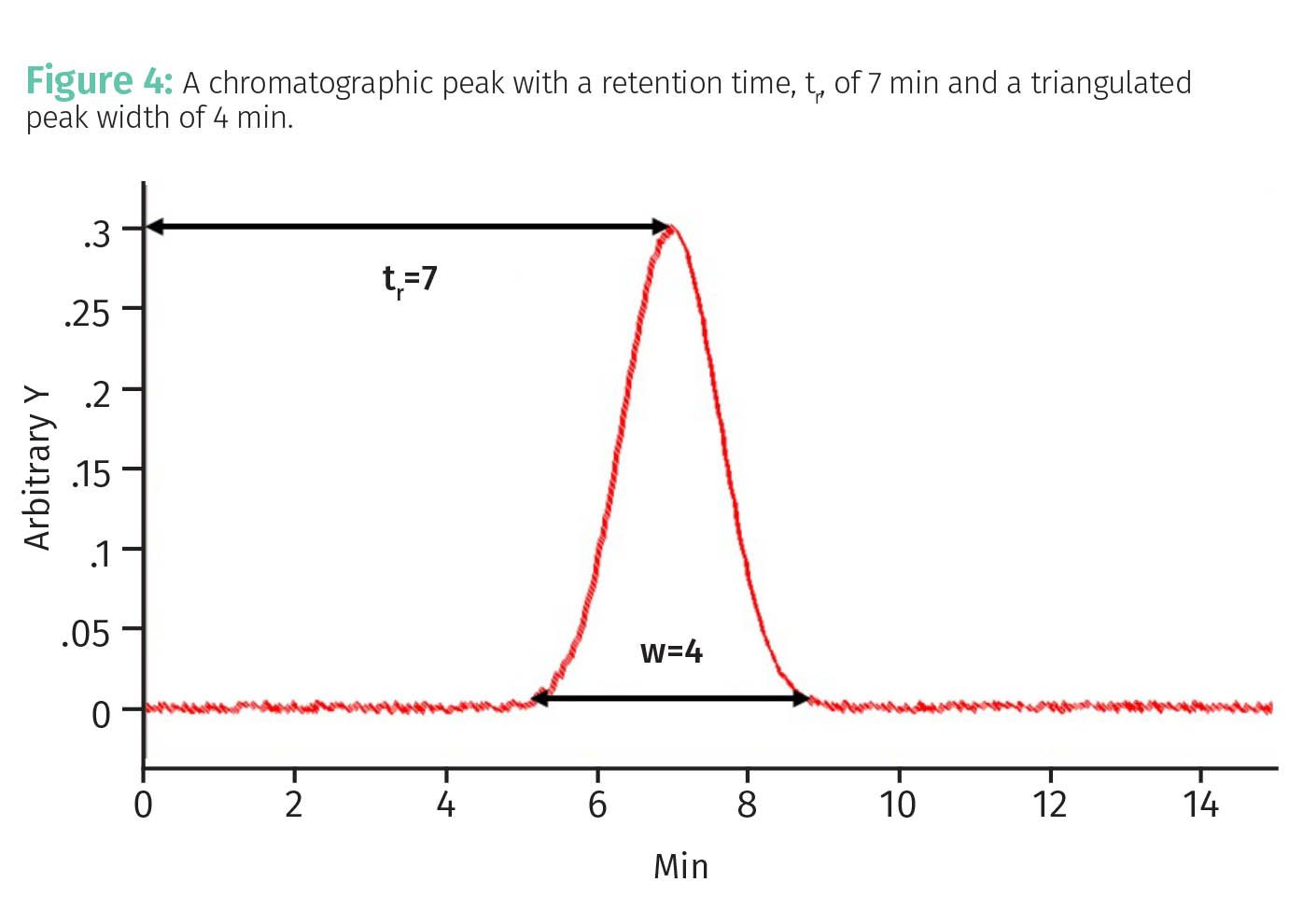
In this figure the retention time of our analyte, t_r, is 7 min and the triangulated peak width w is 4 min. A measure of band broadening for a peak like this is called the plate number which is given by Equation 4:

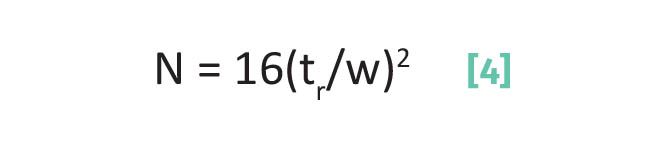
where N is the plate number, t_r is the retention time for a chromatographic peak, and w is the chromatographic peak width measured with the triangulation method.

Note in Equation 4 that both t_r and w are measured in units of time, and that when we divide them the plate number N becomes a unitless quantity. For the peak seen in Figure 4, the plate number is found through Equations 5 and 6:

Now, Equation 4 contains the quantity tr/w, but this is all easier to understand if we think about its inverse, w/t_r. This quantity, the peak width divided by the retention time, measures the percentage of the elution time taken up by the peak width. The more band spreading, the broader the band hence the bigger w/t_r. In Equation 4 then we see the inverse of w/t_r, namely t_r/w, so as band spreading, and hence peak width, goes down N goes up. This means we want N to be large for a separation because it means the peak is narrow compared to the retention time of the peak. So, N is yet another measure of chromatographic separation quality.

A grossly oversimplified way of thinking about the plate number N in a chromatographic separation is that it measures the average number of times an individual molecule adsorbs on the stationary phase before it elutes. Thus, for the peak seen in Figure 4 the average molecule adsorbed 49 times. Now, remember that band spreading goes with F_m. The more times an analyte molecule adsorbs on the column, the less time it spends mobile, the less diffusion and hence band spreading and the narrower the peak will be. This is why N is a measure of band spreading and separation quality.

Pulling it All Together

This is the sixth in a series of articles I have written on chromatographic theory (1,6-9). Across these articles we have derived a number of quantities that I have claimed are measures of chromatographic separation quality including the capacity factor k (6), the separation factor α (9), and now here the plate number N. Given all these quantities how do we get the truest measure of separation quality? Well, there has been a method to my madness. What we ultimately need to do is discover what I will call the master resolution equation for chromatography because it includes k, α, and N. Because the master resolution contains these three variables, we had to define them and discuss them thoroughly before introducing this equation. The master resolution equation is the best summary of how to measure separation quality and to determine what variables to adjust in what way to optimize a separation. More on this next time.

Conclusion

Chromatographic peaks have both peak heights and widths. Narrower peaks are preferred because it is easier to obtain baseline separation. Wide peaks are a result of band spreading, which is caused by the physical phenomenon of diffusion. More diffusion—hence band spreading—takes place the longer an analyte is in the mobile phase. The plate number, N, is a measure of band spreading because it is related to the number of times an analyte molecule adsorbs on the stationary phase, which means it spends less time mobile and hence less time diffusing.

References

1. Smith, B., Chromatography Theory, Part III: Calculating Elution Times and Capacity Factors, Cannabis Science and Technology, 2023, 6(4), 8-11.
2. Skoog, D.A., West, D.M., and Holler, E.J., Analytical Chemistry: An Introduction, Saunders College Publishing, 1994, 6th Edition.
3. Robinson, J.W., Undergraduate Instrumental Analysis, Marcel Dekker, 1995, 5th Edition.
4. Peter, D., Hayes, J., and Hieftje, G., Chemical Separations and Measurements, W.B. Saunders Co., 1974.
5. Diffusion, https://en.wikipedia.org/wiki/Diffusion.
6. Smith, B., Chromatography Theory, Part III: Calculating Elution Times and Capacity Factors, Cannabis Science and Technology, 2023, 6(2), 8-11.
7. Smith, B., Chromatography Theory, Part II: How Separations Occur Illustrated and Quantitated, Cannabis Science and Technology, 2023, 6(1), 7-12.
8. Smith, B., Principles of Chromatography, Part I: Theory, Cannabis Science and Technology, 2022, 5(9), 8-11.
9. Smith, B., Chromatographic Theory, Part IV: Measuring Separation Quality with Capacity and Separation Factors, Cannabis Science and Technology, 2023, 6(3), 8-11.

About the Columnist

Brian C. Smith, PhD, is Founder, CEO, and Chief Technical Officer of Big Sur Scientific. He is the inventor of the BSS series of patented mid-infrared based cannabis analyzers. Dr. Smith has done pioneering research and published numerous peer-reviewed papers on the application of mid-infrared spectroscopy to cannabis analysis, and sits on the editorial board of Cannabis Science and Technology. He has worked as a laboratory director for a cannabis extractor, as an analytical chemist for Waters Associates and PerkinElmer, and as an analytical instrument salesperson. He has more than 30 years of experience in chemical analysis and has written three books on the subject. Dr. Smith earned his PhD on physical chemistry from Dartmouth College.
Direct correspondence to: brian@bigsurscientific.com

How to Cite this Article:

Smith, B., Chromatographic Theory, Part VI: Peak Widths, Band Spreading, and Plate Number, Cannabis Science and Technology, 2023, 6(5), 6-9.