Chromatographic Theory, Part VII: The Master Chromatographic Resolution Equation

In the last six columns (1-6) I have presented three quantities that were used to measure chromatographic separation quality, the capacity factor, the separation factor, and the number of plates. Here, we finally pull all this together into what is called the Master Resolution equation for chromatography. This equation combines all three measures of separation quality, and an understanding of these parameters will go a long way towards allowing you to rationally develop chromatographic methods.

Review of Capacity Factor, Separation Factor, and Number of Plates

Capacity Factors

Recall (3) that the capacity factor for a chromatographic peak is calculated from its elution time and the void time as given by Equation 1a:

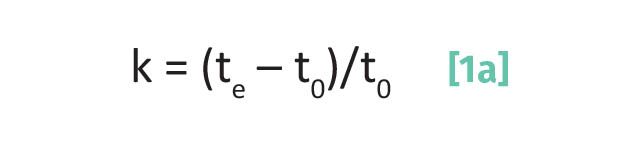
where k is the capacity factor, t_e is the elution time, and t₀ is the void time.

Note that since both the numerator and denominator in equation 1 are in units of time, the units of the capacity factor are unitless.

A way of thinking about the capacity factor, which I perhaps should have mentioned in our earlier discussion (3), is that it is a measure of the affinity for or the strength of the interaction of analyte molecules with the stationary phase. Consider trying to separate a mixture of ethanol and toluene. As a reminder, their chemical structures are shown in Figure 1, with toluene on the left and ethanol to the right.

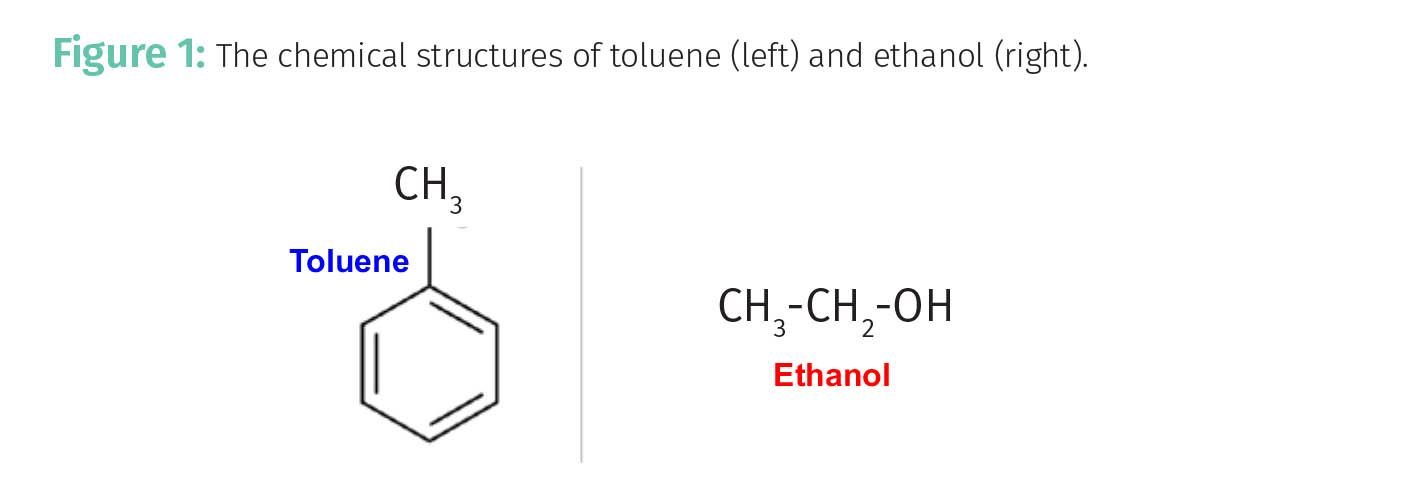
Imagine we are performing liquid chromatography with a silica stationary phase, which has a large number of polar Si-OH groups on the surface, and a non-polar mobile phase such as hexane. Recall (1-6) that the elution time is determined in part by F_s, the fraction of time an analyte molecule spends adsorbed on the stationary phase as seen in Equation 1b.

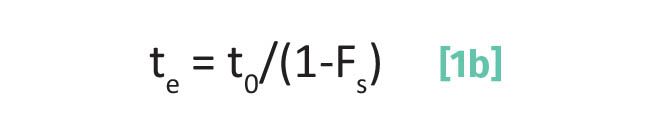
where t_e is the elution time, t_o is the void time, and F_s is the fraction of time spent adsorbed.

A non-polar molecule like toluene will have low affinity for the polar stationary phase, a high affinity for the non-polar mobile phase, have a low value of F_s, and elute early. Whereas a polar molecule like ethanol, will have a high affinity for the polar stationary phase, a low affinity for the non-polar mobile phase, have a larger value of F_s, and elute later. The difference in F_s values and hence elution times for these two analytes leads them to have two different capacity factors as illustrated by the chromatogram in Figure 2.

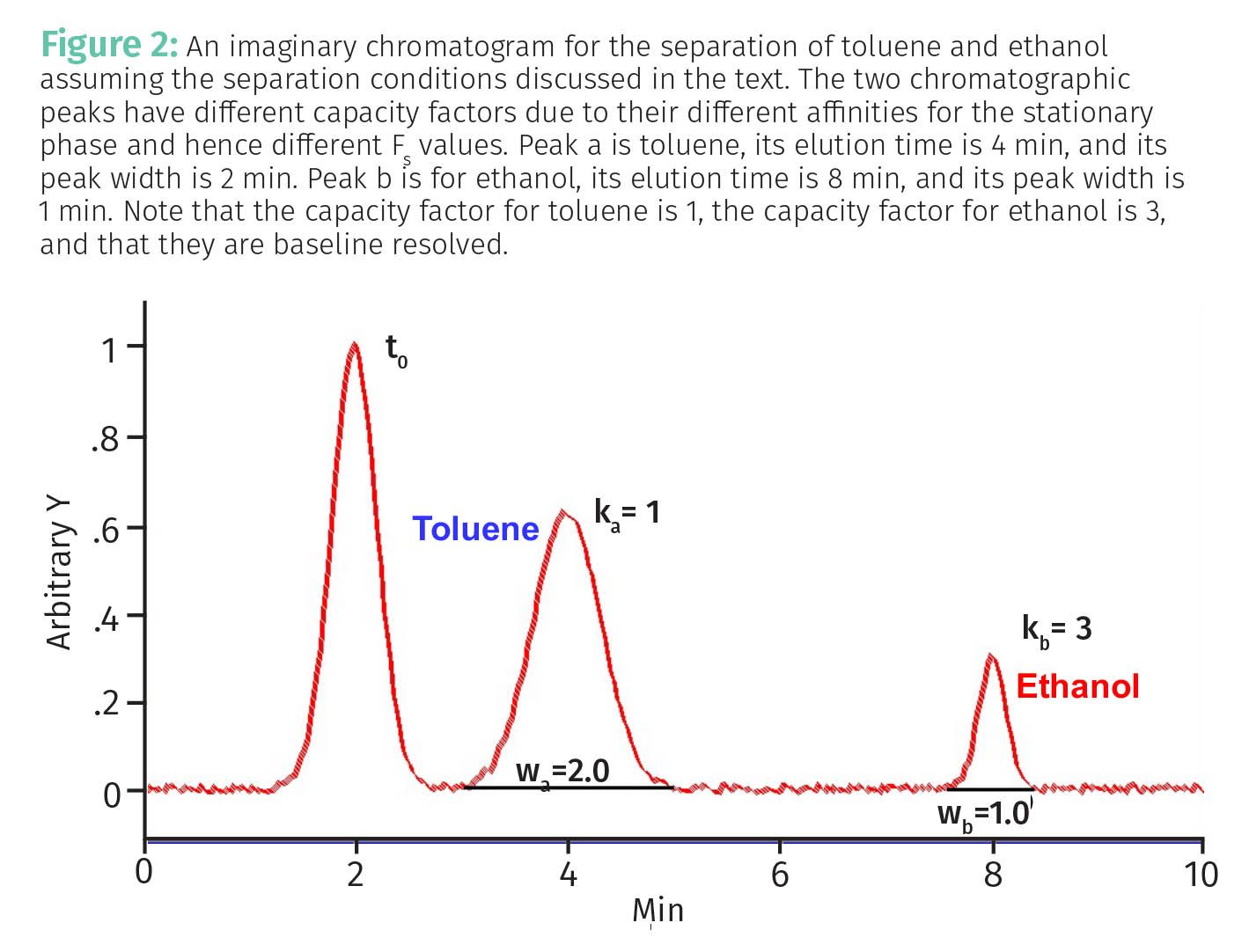
In Figure 2 we can imagine toluene elutes first with a capacity factor of 1, while ethanol elutes later with a capacity factor of 3. Guess what, we have finally used all the chromatographic theory we have learned to understand a real world separation!

Finally, recall (3) that the capacity factor resets the x-axis scale on a chromatogram into void time units. The capacity factor measures how many void times after t₀ an analyte elutes. Thus, in Figure 2 toluene elutes 1 void time after t₀, while ethanol elutes 3 void times after t₀. Also, remember that calculating the capacity factors for a separation helps us compare elution times across separations taken under different conditions, such as at different flow rates (3). Versatile thing that capacity factor, isn’t it?

Separation Factors

The separation factor for a pair of chromatographic peaks is given by Equation 2 (4):

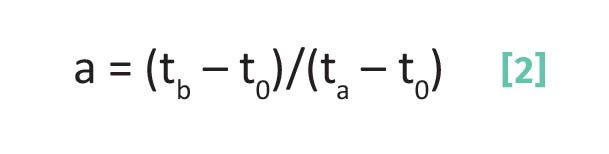
where a is the separation factor, t_b is the retention time of peak b, t_a is the retention time of peak a, t₀ is the void time, and t_b > t_a.

The restriction in Equation 2 that the retention time of peak b be greater than peak a exists so that the separation factor is a number >=1. Since in Equation 2 the numerator and denominator are both in units of time, like the capacity factor the separation factor is a unitless quantity. Above we saw that the capacity factor was a calculation for a specific chromatographic peak. Whereas, note that the separation factor is for a pair of chromatographic peaks and is a measure of how well two peaks are separated from each other.

We found previously (4) that we can derive that the separation factor for a pair of peaks can be calculated from the ratio of their capacity factors as seen in Equation 3:

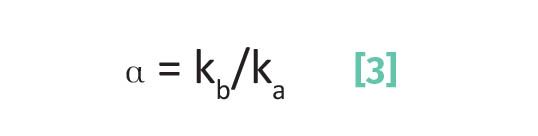
where k_a is the capacity factor for analyte a and k_b is the capacity factor for analyte b.

Thus, for the separation in Figure 2, the separation factor is 3/1 or 3. Note this is an excellent, baseline resolved separation. Note that in Figure 3 α for the two peaks is also 3, even though this is a poor separation. This illustrates the problem that separation factors ignore peak widths.

Chromatographic Resolution

Recall (5) that the separation factor as a measure of chromatographic quality falls apart when peaks are wide. We found (5) that a quantity called the chromatographic resolution gets around this problem by including peak position and width as a measure of separation quality as given by Equation 4:

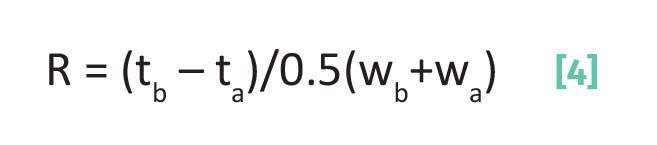
where R is the resolution, t_b is the elution time for peak b, t_a is the elution time for peak a, w_a is the width of peak a, w_b is the width of peak b, and with t_b > t_a.

Note in Equation 4 that both the numerator and denominator are in units of time, so the chromatographic resolution, R, is unitless. If two peaks elute at the same time the numerator in Equation 4, (t_b – t_a), is zero and R is zero. This makes sense since in this case there is no separation. The further apart two peaks are the greater (t_b – t_a) and R goes up. The wider two peaks are the greater (w_b+w_a) and R goes down. Thus, to maximize R we need narrow peaks that are far apart. Using the chromatogram seen in Figure 2, R = (8- 4)/0.5(1+2) = 2.67. Note that these two peaks are baseline resolved.

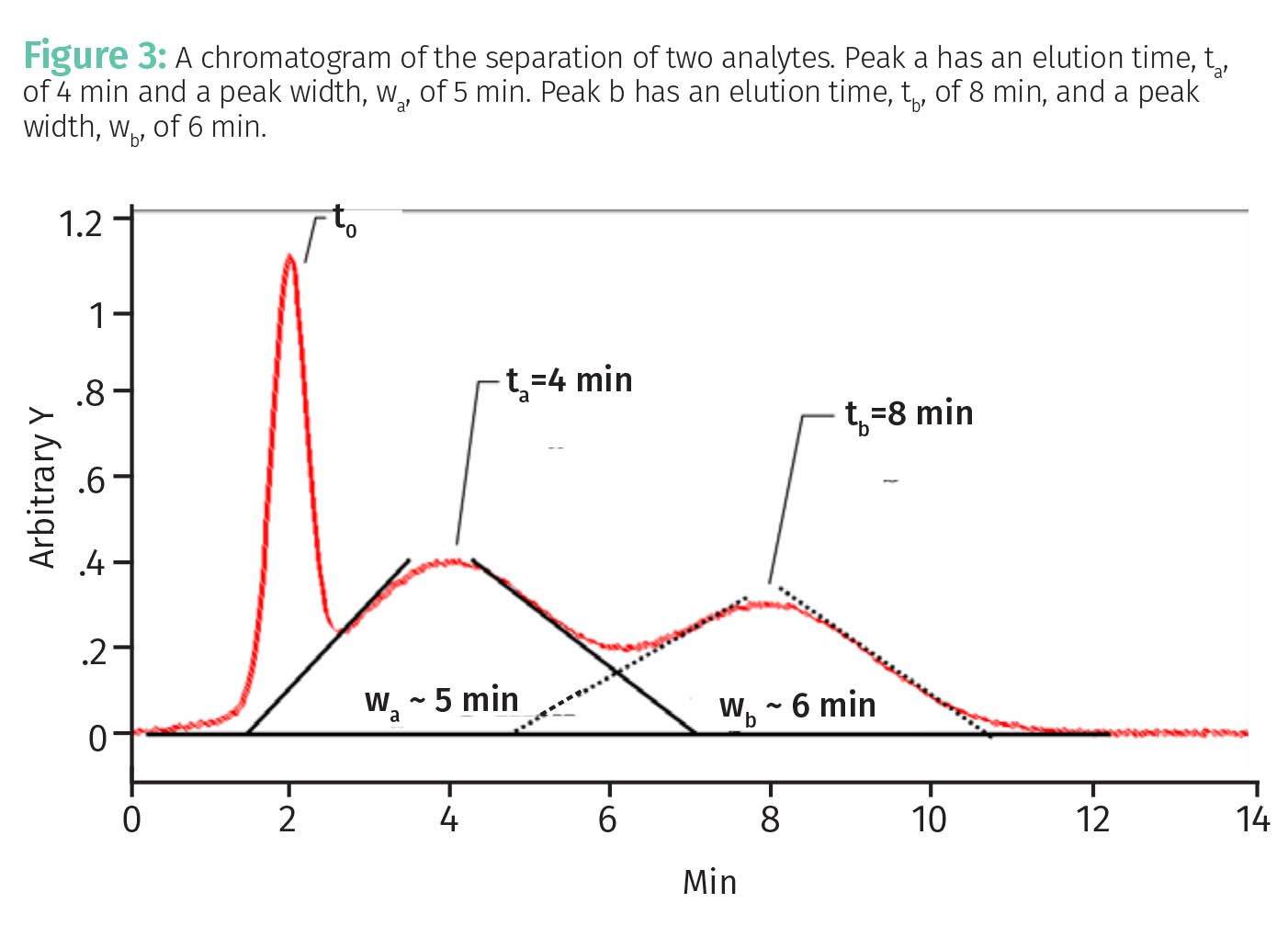
Figure 3 shows a chromatogram where the peaks are neither broad nor far apart.

Using the data in Figure 3 we can calculate for this separation that R = (8-4)/0.5(5+6) = 0.73. Note that these peaks are not baseline resolved and that this is a poor separation. An R value of >1.5 is needed for baseline separation, which should always be the goal of a chromatographic separation. The fact that R gives a good measure of separation quality even in the presence of broad peaks makes it perhaps the best measure of separation quality (5,6).

Plate Number

Finally as we learned last time (6), peak widths are caused, amongst other things, by molecular diffusion while analytes are in the mobile phase. The plate number, N, is measure of peak broadening as seen in Equation 5:

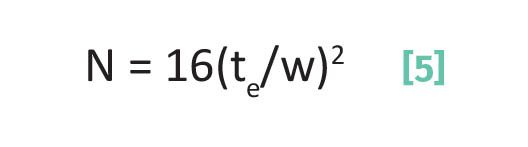
where N is the plate number, t_e is the elution time for a chromatographic peak, and w is the chromatographic peak width measured with the triangulation method.

Recall (6) that N is, in a sense, a measure of the number of times an analyte molecule adsorbs as it works its way down a chromatographic column. The idea is that the more times a molecule adsorbs, the less time it spends mobile where it can diffuse and cause peak broadening. Note in Equation 5 that as t_e goes up so does N, which makes sense since the more times a molecule adsorbs the slower it will be and the later it will elute. Also note from Equation 5, that as peak width goes up N goes down. This makes sense since molecules that do not adsorb often spend a greater fraction of their time mobile hence diffuse a lot leading to peak broadening.

Using the data in Figure 2, N_a, the number of plates for analyte a is 64, while N_b, the number of plates for analyte b is 1024. This means on average molecules of a adsorbed much more often than molecules of b, which is why b elutes later than a. Using the data in Figure 3, N_a = 10 and N_b= 28, which makes sense because in the separation shown in Figure 3 both analytes did not adsorb many times, spent a large fraction of their time mobile, diffused a lot, and hence gave broad peaks.

The Master Chromatographic Resolution Equation

Over the last several months we have covered a lot of chromatographic theory (1-6), but there has been a method to my madness. All these columns were laying the foundation for what we are about to discuss here, the Master Chromatographic Resolution equation (7-11). This equation pulls together in one place everything we have learned about how to understand and quantify chromatographic separations. The full derivation of this equation is beyond the scope of these columns (7-11), but here is this important equation in its final form which can be seen in Equation 6:

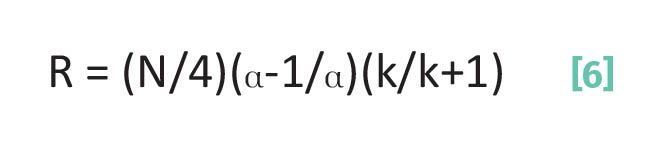
where R is the resolution, N is the plate number, α is the separation factor, and k is the capacity factor.

Recalling that the resolution, R, is our best measure of the quality of a separation. There are three separate terms that R depends upon in equation 6 involving the plate number, separation factor, and capacity factor. The good news is that all the terms in equation 6 are things we have seen before (1-6). The bad news is that there is a lot to unpack here, and it will take at least two columns to do so.

The first term in equation 6 is the square root of the plate number divided by four. Since N is in the denominator here, as N goes up R goes up. That is, the greater the plate number the better the separation. Recall from above that N is a measure of how many times an analyte molecule adsorbs during a separation. The more times a molecule adsorbs, the smaller the fraction of time it spends mobile, the less band spreading, and the narrower the peak and the better the resolution. On the other hand, the fewer times a molecule adsorbs, the fraction of time the molecule spends mobile increases, band spreading goes up, and R goes down. We saw this earlier in Figures 2 and 3.

The second term in equation 6 involves the separation factor, (α-1/α). As this term increases, R goes up. For example, if a = 2 then (α-1/α) = ½, and if α = 4 then (α-1/α) = ¾. This makes sense because as we have seen, the further apart two peaks are the greater their separation factor (4).

The third term in Equation 6 involves the capacity factor and is (k/k+1). Like the separation factor, as k goes up R goes up. For example, if k = 2 then (k/k+1) = 2/3, whereas if k =4 then (k/k+1) = 4/5. This makes sense since k measures elution time in column volumes, and the greater k is the better it is separated from things that elute earlier.

There is a lot more to say about the Master Resolution equation, but there is so much to say it deserves its own column. So, happy separating until next time.

Conclusions

We reviewed concepts previously developed for capacity factor, separation factor, and plate number. I then presented the Master Resolution equation, that shows that to optimize a chromatographic separation we want N, α, and k to be maximized.

References

1. Smith, B., Principles of Chromatography, Part I: Theory Cannabis Science and Technology®, 2022, 5(9), 8-11.
2. Smith, B., Chromatography Theory, Part II: How Separations Occur Illustrated and Quantitated, Cannabis Science and Technology®, 2023, 6(1), 7-12.
3. Smith, B., Chromatography Theory, Part III: Calculating Elution Times and Capacity Factors, Cannabis Science and Technology, 2023, 6(2), 8-11.
4. Smith, B., Chromatographic Theory, Part IV: Measuring Separation Quality with Capacity and Separation Factors, Cannabis Science and Technology, 2023, 6(3), 8-11.
5. Smith, B., Chromatographic Theory, Part V: Chromatographic Resolution, Cannabis Science and Technology, 2023, 6(4), 8-11.
6. Smith, B., Chromatographic Theory, Part VI: Peak Widths, Band Spreading, and Plate Number, Cannabis Science and Technology, 2023, 6(5), 6-9.
7. Skoog, D.A., West, D.M., and Holler, E.J., Analytical Chemistry: An Introduction, Saunders College Publishing, 1994, 6th Edition.
8. Robinson, J.W., Undergraduate Instrumental Analysis, Marcel Dekker,1995, 5th Edition.
9. Peter, D., Hayes, J., and Hieftje, G., Chemical Separations and Measurements, W.B. Saunders Co., 1974.
10. Bayne, S., Carlin, M., Forensic Applications of High Performance Liquid Chromatography, CRC Press, 2010.
11. Carlin, M., Dean, J., Forensic Applications of Gas Chromatography, CRC Press, 2013.

About the Columnist

Brian C. Smith, PhD, is Founder, CEO, and Chief Technical Officer of Big Sur Scientific. He is the inventor of the BSS series of patented mid-infrared based cannabis analyzers. Dr. Smith has done pioneering research and published numerous peer-reviewed papers on the application of mid-infrared spectroscopy to cannabis analysis, and sits on the editorial board of Cannabis Science and Technology. He has worked as a laboratory director for a cannabis extractor, as an analytical chemist for Waters Associates and PerkinElmer, and as an analytical instrument salesperson. He has more than 30 years of experience in chemical analysis and has written three books on the subject. Dr. Smith earned his PhD on physical chemistry from Dartmouth College.
Direct correspondence to: brian@bigsurscientific.com.

How to Cite This Article

Smith, B., Chromatographic Theory, Part VII: The Master Chromatographic Resolution Equation, Cannabis Science and Technology, 2023, 6(6), 7-11.