The Most Important Equation in Potency Analysis is Beer’s Law: An Introduction

Whether you are using high performance liquid chromatography (HPLC) or infrared spectroscopy to measure potency in cannabis samples, you are using Beer’s Law. Beer’s Law relates the amount of light absorbed by a sample to the concentration of molecules in that sample. This equation has a long history of use in analytical chemistry and is used in laboratories around the world every day to perform quantitative analyses. In this introduction to Beer’s Law, I will review its use, history, and begin to cover the theory behind the equation.

When I started this column series in the fall of 2018, my intent was to cover the basics of analytical chemistry for an audience that might be new to the topic. However, as you may have noticed, I have observed a lot of deficiencies in the quality and quantity of testing in the cannabis industry. Therefore, I have devoted a number of columns to railing against these problems and have used this platform to passionately advocate for more and better cannabis testing. Frankly, I have run out of things to rail against. The industry knows how I feel about a number of topics and pointing out the same problems and solutions over and over in column after column is pointless. Hence, it is time to return to the original mission of this column: analytical chemistry. Here and in a few upcoming columns I will introduce you to Beer’s Law, the most important equation in cannabis potency analysis.

What is Beer’s Law?

A little history first. Beer’s Law is sometimes called the Beer-Lambert Law or the Beer-Lambert-Bouguer Law (1). Long story short, Beer, Lambert, and Bouguer all contributed to our understanding of the relationship between the amount of light absorbed by a sample, analyte concentration, and sample thickness, and all three are deserving of having their name appended to this law. However, for the sake of convenience I will use the term “Beer’s Law” for short going forward. And yes, over the years in the analytical chemistry courses I have taught I have had smug students comment on how they “study Beer’s Law every night.” To be clear, the Beer in Beer’s Law is named after a guy, not the intoxicating beverage.

Since we are speaking of light absorbance here, Beer’s Law relates to the science of spectroscopy, which is the study of the interaction of light with matter (2,3). To measure the amount of light absorbed by a sample we use an instrument called a spectrometer, which measures a spectrum, which is a plot of light intensity on the y-axis versus some property of light on the x-axis (2). Typical x-axis units used in spectra include microns, nanometers (nm), and wavenumber (cm-1), and typical y-axis units used in spectra include absorbance and percent transmittance (%T) (2). People who practice the art of spectroscopy are called spectroscopists, and as you may have figured out from my past columns extolling the virtues of infrared spectroscopy for potency analysis, your author is a spectroscopist—which is a difficult profession to explain to people at cocktail parties.

Since Beer’s Law relates the amount of light absorbed by a sample to the concentration of molecules (technically I should say “chemical species” here, but for the purposes of cannabis potency analysis we are detecting molecules) in that sample, Beer’s Law is what makes spectroscopy quantitative. It is then true to say that spectroscopy is a quantitative technique. Since Beer’s Law involves spectroscopy, how is Beer’s Law relevant for potency analysis? First, there exist potency analyzers that use infrared spectroscopy to measure potency in cannabis biomass, extracts, distillates, and tinctures (4–9). Second, most high performance liquid chromatography (HPLC) potency methods use ultraviolet-visible (UV-vis) spectroscopy detection (10,11), which uses Beer’s Law to measure the amount of light absorbed by cannabinoids in solution as they leave an HPLC column. Please note here that Beer’s Law works regardless of the wavelength used. Thus, it is incorrect to say as some chromatographers have asserted to me, that UV-vis spectroscopy is quantitative while infrared spectroscopy is not. Beer’s Law can work at any wavelength.

A typical Beer’s Law experimental set up is illustrated in Figure 1 (3).

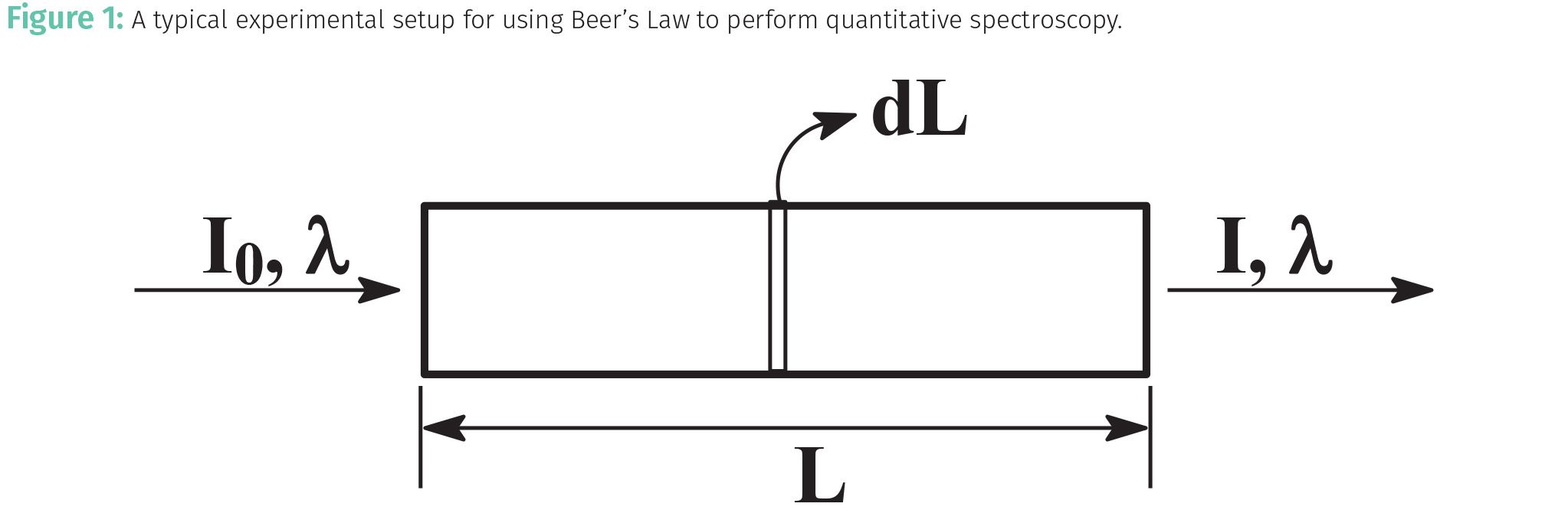
To the left in Figure 1 a beam of monochromatic (single wavelength) light with wavelength λ and intensity I₀ is impinging upon a sample of thickness L. Spectroscopists call the sample thickness that a light beam passes through the pathlength. To the right in Figure 1, we see the light beam leaving the sample still with wavelength λ but now with intensity I. The intensity of the light beam has decreased because some of the light has been absorbed by the sample. The setup in Figure 1 is referred to by spectroscopists as a transmission experiment, which means the light passes through the sample, and it is the light that has been transmitted through the sample that is focused upon the spectrometer's detector to measure a spectrum.

Beer’s Law has the following form (3), found in equation 1:

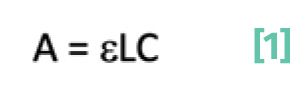
where A is the absorbance; Ɛ is the absorptivity; L is the pathlength; and C is the concentration.

Absorbance is a unitless quantity that measures the amount of light absorbed by a sample. It is calculated from the following equation 2:

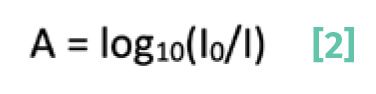
where A is the absorbance; I₀ is the measured light intensity before the light beam enters a sample; and I is the measured light intensity after the light beam has exited a sample.

The nature of I and I₀ are illustrated in Figure 1. Note that equation 2 contains a base 10 logarithm. Also note that since equation 2 contains the ratio I₀/I and because I is the denominator, the less light leaving the sample the higher the absorbance. Again, the sample absorbing light causes less of it to leave the sample than entered it.

The absorptivity, Ɛ , is the proportionality constant between absorbance and concentration. It is a fundamental physical constant for a pure molecule at a given wavelength, temperature, and pressure. For example, the absorptivity of pure acetone at 1715 cm^-1, 25 °C, and 1 atmosphere of pressure is a molecular constant for acetone. This absorptivity is, in effect, a measure of how much light a sample of pure acetone will absorb under standard conditions at a given wavenumber, which has been found to be a constant. Note that since Ɛ depends upon wavenumber, this quantity will vary for the same molecule. For instance, the absorptivity of acetone at 1715 cm^-1 is not the same as for acetone at 1220 cm^-1. Also, the absorptivity can vary from molecule to molecule. Hence, the absorptivity of acetone at 1715 cm^-1 is different than that of water at 1715 cm^-1. This is all I will say about the absorptivity for now, but I will have a lot more to say about it in upcoming columns.

As mentioned above, L is the pathlength or thickness of the sample seen by the light beam. The concentration, C, is typically in units of moles/liter (moles per liter). These units are fine for analyzing liquids, but it is often hard to know the moles/liter for solids. In this case, we often use weight percent in Beer’s Law instead. Weight percent is the unit typically used in cannabis potency analyses.

Since the absorbance is a unitless quantity, when the units of the absorptivity, pathlength, and concentration are multiplied together they must cancel. How does this happen? The units of the concentration are in moles/volume. Remember that volume has units of width x height x width, which is length cubed, or L³. The units of the pathlength are simply length. When we multiply the units out in Beer’s Law the results are seen in equation 3:

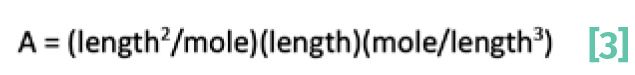
Since the product of (length)(mole/length³) is mole/length², the units of the absorptivity must thus be length²/mole to cancel to make A unitless. The absorptivities’ units of length²/mole may seem physically counterintuitive. Length² is an area, and a mole measures number of molecules. How can a molecule have a property that is units of area per amount of stuff? As promised above, all will be revealed about the mysterious absorptivity in a future column.

Building Calibrations with Beer’s Law

The point of Beer’s Law is that by using it to build a calibration, we can use spectroscopic absorbance measurements to predict the concentration of analytes in an unknown sample. Why, you may ask, would one use spectroscopy instead of chromatography for cannabis potency analysis? Because as I pointed out in numerous peer-reviewed papers, spectroscopy will always be faster, cheaper, and easier than chromatography (4-9). The first step in building a spectroscopic calibration is to generate standards, which are samples with known amounts of the analytes of interest in them. For HPLC, where the analyte is extracted from the sample into a solution, certified standards of known amounts of the analyte in solution are used to build calibrations. For infrared spectroscopy the samples are normally analyzed in the sample matrix as is without extraction. In this case to build a calibration you need matrix reference materials, which are samples of the actual sample matrix with known amounts of the analyte in them. In this case, the known concentrations in matrix reference materials can be determined, for example, by gas or liquid chromatography.

To determine the concentration of analyte in an unknown sample we can re-arrange equation 1 to solve for the concentration of analyte in the unknown sample, C_unk, as seen in equation 4:

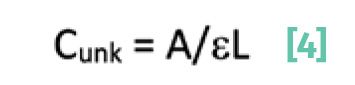
where all the quantities appearing in equation 4 have been previously defined.

Thus, to determine the concentration of analyte in our unknown, C_unk, we need to know its pathlength, absorptivity, and absorbance. The pathlength is usually a known quantity, for example it is the thickness of a sample cell for liquids, such as used in UV-vis detectors for HPLC. The absorbance of the unknown sample is what we measure with a spectrometer, so the only quantity left that we need to find to predict concentration in unknown samples is the absorptivity.

The entire purpose of building a spectroscopic calibration is to determine the absorptivity for the analyte of interest, at the wavelength of interest, in the sample matrix of interest. For simple single analyte Beer’s Law quantitative determination, the calibration process is as follows:

1. Generate standards with known amounts of analyte in the matrix
   of interest.
2. Measure the absorbance of the standards at the wavelength of interest using a spectrometer. This will typically be a peak height or area (more on this in later columns).
3. Plot the measured absorbance versus the known concentration of analyte in the standards. This is known as a Beer’s Law Plot.
4. Beer’s Law has the form of a straight line. A comparison of Beer’s Law and the equation for a straight line (3) is seen in
   equation 5:

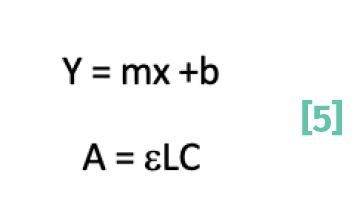
where Y is the y-axis data value; m is the slope of a line; x is the x-axis data value; and b is the y-intercept.

For a Beer’s Law plot, Y would be the measured absorbance, X would be the known concentration, and the slope would be ƐL. Ideally, a plot of absorbance versus concentration produces a straight line, from which the slope and hence ƐL can be calculated.

An example of a real-world Beer’s Law plot, which I will call a calibration line going forward, is seen in Figure 2 (3).

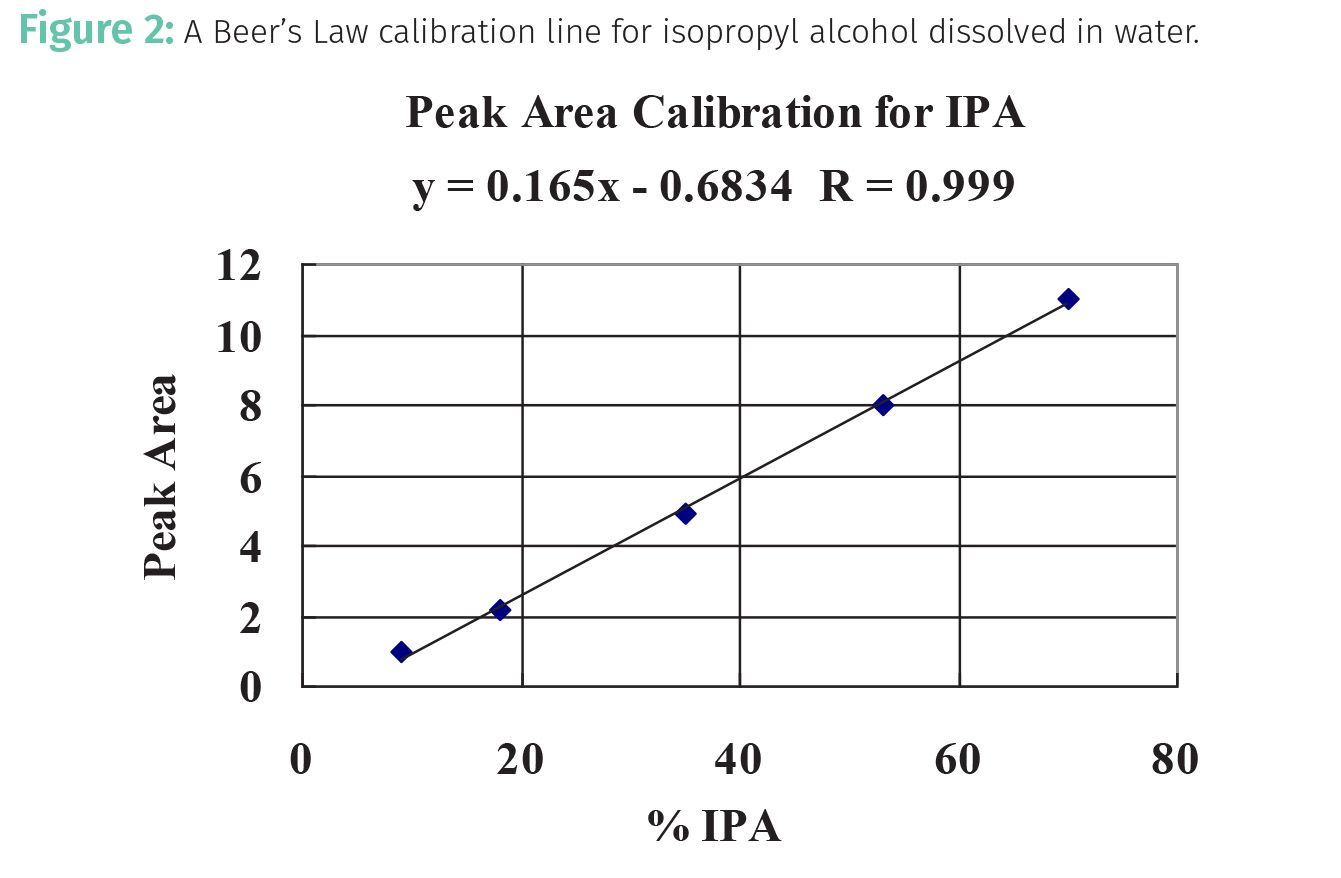
The matrix of interest is isopropyl alcohol (also known as IPA or rubbing alcohol) dissolved in water, the analyte of interest is IPA, and the absorbance data plotted in Figure 2 was determined by the peak height at 2973 cm-1 as illustrated in Figure 3.

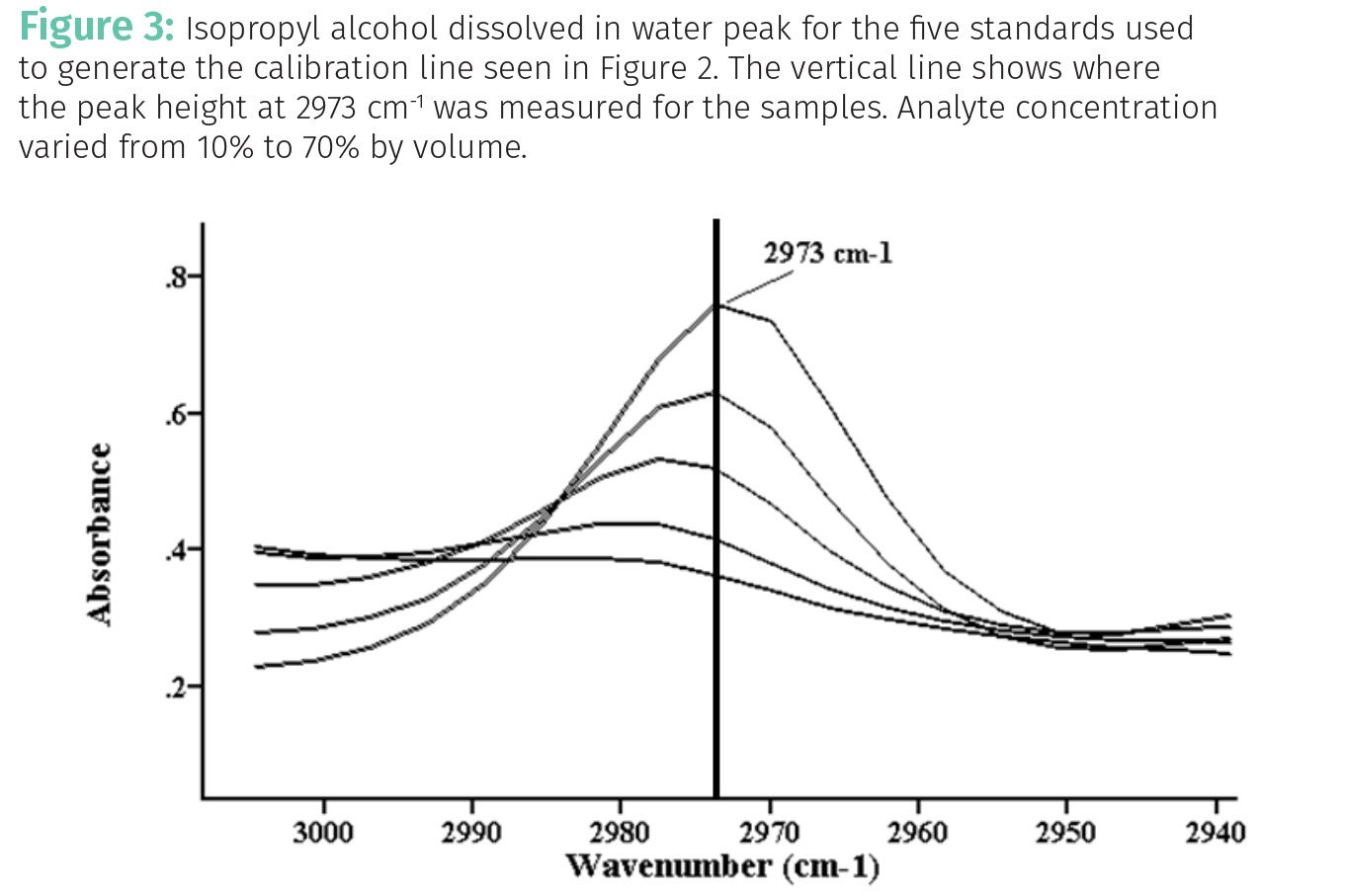
Figure 3 shows the spectra of 5 IPA in water standards in the 3000–2940 cm^-1 range, and the vertical line represents where the peak height at 2973 cm^-1 was measured for the five samples. As you can see, the absorbance (peak height) increases from small to large across the five samples as the concentration of IPA in the samples increased.

The equation of the calibration line in Figure 3 is seen at the top and is equation 6:

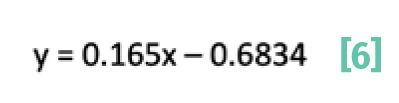
where the slope is 0.165. This is the ƐL value that we would use in equation 4 along with the measured absorbance to determine the concentration of analyte in an unknown sample. A measure of calibration quality is called the correlation coefficient and is measured on a zero to one scale. For a calibration line like that seen in Figure 2, a figure of one would be a perfect calibration where all the data points fall dead center on a straight line. This of course never happens, but the correlation coefficient for the calibration line in Figure 2 listed at the top and has a value of 0.999, very close the perfect score of 1. This is your final proof that spectroscopy is a quantitative technique.

Conclusions

Beer’s Law is used to perform quantitative spectroscopic analyses and relates the amount of light absorbed by a sample to the concentration of analytes in that sample. This equation is used by both infrared spectroscopy and HPLC to measure cannabinoids in cannabis samples. The details behind Beer’s Law were presented, how to obtain Beer’s Law calibration lines, and how to use these lines to determine concentrations in unknown samples were discussed.

References

1. https://en.wikipedia.org/wiki/Beer–Lambert_law.
2. B.C. Smith, Fundamentals of Fourier Transform Infrared Spectroscopy 2nd Ed. (CRC Press, Boca Raton, Florida, 2011).
3. B.C. Smith, Quantitative Spectroscopy: Theory and Practice, (Elsevier, Boston, Massachusetts, 2002).
4. B.C. Smith, M. Lewis, and J. Mendez, “Optimization of Cannabis Grows Using Fourier Transform Mid-Infrared Spectroscopy,” PerkinElmer Application Note (2016).
5. B.C. Smith, Cannabis Science and Technology 2(6), 28-33 (2019).
6. B.C. Smith, Cannabis Science and Technology 3(6), 24-38 (2020).
7. B.C. Smith, Terpenes and Testing Jan.-Feb. 2018., Pg. 32.
8. Brian C. Smith, Terpenes and Testing Nov.-Dec. 2017, pg. 48.
9. B.C. Smith., C.A. Fucetola, K. Ehrmantraut, and T. Hagan, Terpenes and Testing Sept./Oct. 2020, Pages 19-24.
10. M.W. Giese, M.A. Lewis, L. Giese, and K.M. Smith, Journal of AOAC International 98(6), 1503 (2015).
11. C. Giroud, CHIMIA Intl. Journal of Chemistry 56, 80 (2002).

About the Columnist

Brian C. Smith, PhD, is Founder, CEO, and Chief Technical Officer of Big Sur Scientific. He is the inventor of the BSS series of patented mid-infrared based cannabis analyzers. Dr. Smith has done pioneering research and published numerous peer-reviewed papers on the application of mid-infrared spectroscopy to cannabis analysis, and sits on the editorial board of Cannabis Science and Technology®. He has worked as a laboratory director for a cannabis extractor, as an analytical chemist for Waters Associates and PerkinElmer, and as an analytical instrument salesperson. He has more than 30 years of experience in chemical analysis and has written three books on the subject. Dr. Smith earned his PhD on physical chemistry from Dartmouth College.
Direct correspondence to: brian@bigsurscientific.com

How to cite this article:

B. Smith, Cannabis Science and Technology, 5(2), 10-13 (2022).